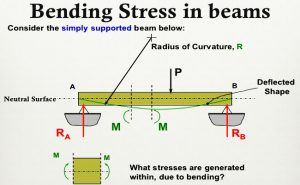
If it’s necessary to calculate the value of bending stresses developed in a loaded beam, count on the two cross-sections of a beam as HE and GF, initially parallel as presented in fig 1(a). While bending the beam, it’s far expected that these sections remain parallel i.e. H’E’ and G’F’, the very last location of the sections, still stay as straight lines and then they subtend some angle q.
Now, assume fiber AB in the material, at a span y from the N.A, if the beam bends, it’ll amplify to A’B’
As CD and C’D’ stay on the neutral axis and it is predicted that the stress on the neutral axis is 0. therefore, no strain exists on the neutral axis.
Assume any arbitrary a cross–section of beam, as presented above now the strain on a fibre at a distance ‘y’ from the N.A, is designated with the expression.
Now the term stands for the property of the material and is described as a second moment of area of the cross–section and is presented with a symbol I.
This equation is known as the Bending theory Equation. The above evidence consists of the theory of pure bending with none existence of shear force. So, it is defined as the pure bending equation. This equation presents distribution of stresses that are usual to cross–section i.e. in x-direction.